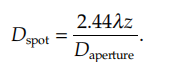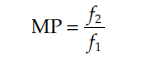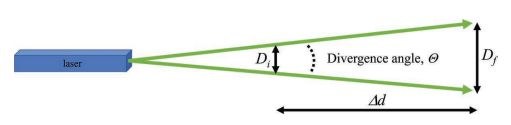
Another characteristic of all lasers is the divergence of the output beam. As a laser beam leaves the output coupler it will spread as it propagates. In other words, the spot diameter will get larger as the beam travels farther from the laser as shown in Figure 6.20. The full-angle beam divergence is calculated by

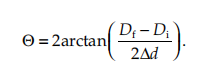
where
Df is the final spot diameter,
Di is the initial spot diameter, and
Δd the distance between the final and initial spots.
Typical divergences for HeNe lasers, for example, are usually measured in the milliradians.

All laser beams diverge naturally as a consequence of propagation and diffraction. Optical elements can be used to focus beams but there are limits to the control of beam divergence simply due to the physics of propagation.

A common practice for laser scientists and engineers is to collimate a laser beam using a telescope or other dispersive optical element system. It is often misunderstood by the novice laser operator that a collimated laser beam will not remain collimated forever because of diffraction.

Figure 6.21 shows a typical configuration for expanding and collimating a laser beam using two lenses and a spatial filter (a pinhole). The two lenses are placed apart from each other such that their focal spots coincide and in the near field within a meter or two from the lenses the beam appears to be perfectly collimated with no divergence. While this may be true (no divergence) diffraction due to propagation is still happening and in the far-field

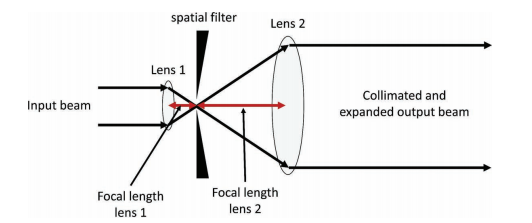
distances (greater than a thousand meters or so) the beam size is limited by the output lens aperture diameter, D aperture, and the distance propagated, z, as

which is the increase in beam diameter from input to output of the optical system. Many laser beams exit the laser aperture in a very tiny beam only a few millimeters in diameter and for many applications this beam needs to be expanded many times. The expansion of the beam expander shown in Figure above is calculated as

where f1 and f2 are the focal lengths of lenses 1 and 2, respectively, and MP is the magnification power of the beam expander. We also should realize that the beam expander can be used in reverse as a beam reducer.